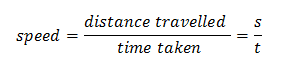Class 9 Physics - 1st chapter MOTION

1. Introduction

• In our day by day life, we see heaps of things moving around for instance vehicle going through from one place to other, individual riding on a bike and a lot increasingly like this. 

• In logical terms an item is said to be in movement ,on the off chance that it changes its situation with the progression of time and on the off chance that it doesn't transform it position with the progression of time, it is said to be very still 

• Both the movement and rest are relative terms for instance versatile kept on the table is resting at its position yet it is moving in the sense as earth is turning on its pivot. So for an individual seeing portable from earth it is very still and for individual on moon earth appears to change its situation with time thus versatile is moving. 

• Least difficult instance of movement is rectilinear movement which is the movement of the article in a straight line 

• In our depiction of article, we will regard the item as a point object. 

• Item under thought can be treated as point object if the extent of the article is a lot littler than the separation gone by it in sensible time span for instance length of an engine vehicle voyaging a separation of 500km can be dismissed w.r.t. separate gone by it. 

2. Motion along a straight line

• The easiest sort of movement is the movement along a straight line. 

• Two distinct amounts Distance and Displacement are utilized to portray the general movement of an item and to find its last position with reference to its underlying position at a given time. 

• Separation in material science, is the length of the way (the line or bend) depicted by an article traveling through space. Separation is free of bearing. In this manner, such physical amounts that don't require course for their total depiction are called scalars. 

• At the point when a body moves starting with one position then onto the next the most brief separation between the underlying and last position of the body alongside its bearing is known as uprooting. Uprooting has both course and size for its entire portrayal and consequently such physical amounts are known as a vectors. 

• The separation gone by a moving body can't be zero yet the last uprooting of a moving body can be zero. 

• In the event that a body covers square with separations in equivalent interims of time, it is said to have uniform movement. 

• In the event that a body covers unequal separations in equivalent interims or equivalent separations in unequal interims at that point body is said be having non-uniform movement.

3. Speed

• Speed is defined as the total distance travelled by the object in the time interval during which the motion takes place. SI unit of speed is meter per second. So,

        where s is the distance travelled by the body and t is the time taken by the body to travel distance "s"

• Speed of a body gives us the thought how moderate or quick that body is moving. 

• The proportion of all out separation to add up to time taken by the body gives its normal speed. The speed of a body at a given moment is its momentary speed. 

• A body is said to have consistent or uniform speed on the off chance that it voyages break even with separation in equivalent interims of time.

4. Velocity

• The rate of change of displacement of a body with the passage of time is known as velocity of the body. Velocity of an object is measured in meter per second in SI units. So,
  
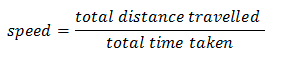
• Velocity is nothing but the speed of an object moving in a definite direction.
• The velocity of an object can be uniform or variable. It can be changed by changing the object�s speed, direction of motion or both.
• So velocity of a body is a vector quantity involving both distance and displacement whereas speed of a body is a scalar quantity and it only has magnitude and does not have specific direction.
• Thus a body is said to be moving with uniform velocity if it covers equal distances in equal intervals of time in a specified direction.
• A body is said to be moving with non uniform velocity if it covers unequal distances in equal intervals of time and vice-versa in a specified direction or if it changes the direction of motion.
• The velocity of a body can be changed in two ways first by changing the speed of the body and second by changing the direction of motion of the body by keeping the speed constant. Also both speed and direction of the body can be varied in order to change the velocity of the body.
• When velocity of the object changes at a uniform rate, then average velocity is given by the arithmetic mean of initial velocity and final velocity for a given period of time. That is,
  
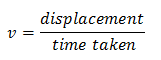
  Where u is the initial velocity of the object and v is the final velocity of the object.

5. acceleration

• Acceleration is a measure of the change in the velocity of an object per unit time and mathematically it is given as

• If the velocity of an object changes from an initial value u to the final value v in time t, the acceleration a is given by,
  a=v−ut
  and this kind of motion is called accelerated motion.
• A body has uniform acceleration if it travels in a straight line and its velocity increases by equal amount in equal intervals of time for example freely falling bodies, motion of ball rolling down the inclined plane etc.
• A body has non uniform acceleration if its velocity increases or decreases by unequal amount in equal intervals of time.
• If acceleration is in the direction of the velocity then it is positive acceleration and if it is in the direction opposite to the direction of velocity then it is negative and the negative acceleration is termed retardation.
• SI unit of acceleration is ms^-2.

6. Equations of uniformly accelerated motion

• There are three equations of bodies moving with uniform acceleration which we can use to solve problems of motion

First Equation of motion

• The first equation of motion is v=u+at , where v is the final velocity and u is the initial velocity of the body.
• First equation of motion gives velocity acquired by body at any time t.
• Now we know that acceleration
  
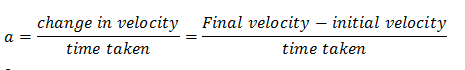
  so, a=v−ut
  and, at=v−u
  rearranging above equation we get first equation of motion that is
  v=u+at

  Second Equation of motion

  • Second equation of motion is
    s=ut+12at2
    where u is initial velocity, a is uniform acceleration and s is the distance travelled by body in time t.
  • Second equation of motion gives distance travelled by a moving body in time t.
  • To obtain second equation of motion consider a body with initial velocity u moving with acceleration a for time t its final velocity at this time be v. If body covered distance s in this time t , then average velocity of the body would be
    
    Distance travelled by the body is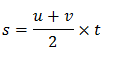
    
    From first equation of motion 
    v=u+at
    So putting first equation of motion in above equation we get ,
    s=u+u+at2×t=(2u+at)t2=2ut+at22
    Rearranging it we get
    s=ut+12at2

  Third equation of motion

  • Third equation of motion is
    v2=u2+2as where u is initial velocity, v is the final velocity, a is uniform acceleration and s is the distance travelled by the body.
  • This equation gives the velocity acquired by the body in travelling a distance s.
  • Third equation of motion can be obtained by eliminating time t between first and second equations of motion.
    So, first and second equations of motion respectively are
    v=u+at and s=ut+12at2
    Rearranging first equation of motion to find time t we get
    t=v−ua
    Putting this value of t in second equation of motion we get
    s=u(v−u)a+12a(v−ua)2
    
    s=uv−u2a+a(v2+u2−2uv)2a2
    
    s=2uv−2u2+v2+u2−2uv2a
    
    Rearranging it we get 
    v2=u2+2as
  • These three equations of motion are used to solve uniformly accelerated motion problems and following three important points should be remembered while solving problems
    1. if a body starts moving from rest its initial velocity u=0
    2. if a body comes to rest i.e., it stops then its final velocity would be v=0
    3. If a body moves with uniform velocity then its acceleration would be zero.

       7. Graphical representation of motion

       • A graph is a pictorial representation of the relation between two sets of data of which one set is of dependent variables and the other set is of independent variables.
       • To describe the motion of an object, we can use line graphs. In this case, line graphs show dependence of one physical quantity, such as distance or velocity, on another quantity, such as time.

       Distance Time Graphs

       • The change in the position of an object with time can be represented on the distance-time graph.
       • In this graph, time is taken along the x-axis and distance is taken along the y-axis.
       • Distance time graphs of a moving body can be used to calculate the speed of the body as they specifically represent velocity.
       • The distance time graph for a body moving at uniform speed is always a straight line as distance travelled by the body is directly proportional to time as shown below in the figure 1.
       • The distance time graph for a body moving with non uniform speed is a curve and is shown below in the figure 2.
         
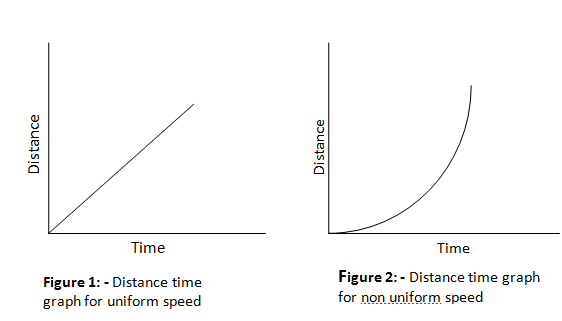
       • The distance time graph is parallel to time axis when the object is at rest and is shown below in figure 3.
         
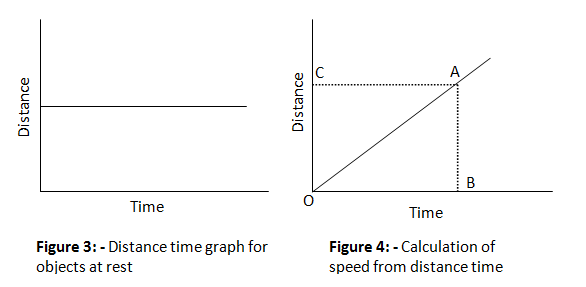
       • To calculate speed of the body from distance time graph say at point A first draw a perpendicular AB on time axis and a perpendicular AC on distance axis so that AB represents the distance travelled by the body in time interval OB and since we know that
         

         Velocity time graphs

         • The variation in velocity with time for an object moving in a straight line can be represented by a velocity-time graph.
         • In this graph, time is represented along the x-axis and the velocity is represented along the y-axis.
         • The product of velocity and time give displacement of an object moving with uniform velocity. The area enclosed by velocity-time graph and the time axis will be equal to the magnitude of the displacement.
         • If a body moves with a constant velocity then velocity time graph for this body would be straight line parallel to time axis as shown below in the figure 5
           
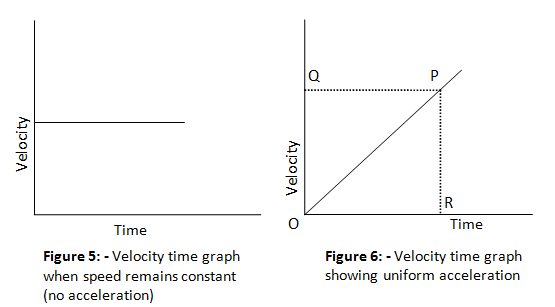
         • The velocity time graph of uniformly changing velocity is shown in figure 6 and is a straight line. We can find out the value of acceleration using the velocity time graph.
         • For calculating acceleration at time corresponding to point R draw a perpendicular RP from point R as shown in figure 6 and we know that 
           
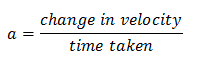
           Here change in velocity is represented by PR and time taken is equal to OR. So,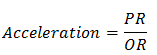 
           
           which is equal to the slope of velocity time graph. So we conclude that slope of velocity time graph of moving body gives its acceleration.
         • The distance travelled by moving body in a given time will be equal to area of triangle OPR as shown in figure 6
           
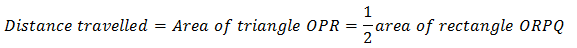
           so,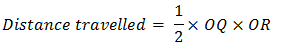
           
         • When the velocity of a body changes in an irregular manner then velocity time graph of the body is a curved line.

           8. Equations of motion by graphical method

           • We already know about equations of motion when an object moves along straight line with uniform acceleration. We already know how to derive them but these equations can also be derived by graphical method.

           a. Equation for velocity time relation

           • Consider the velocity-time graph of an object that moves under uniform acceleration as shown below in the figure 7.
           • From this graph, you can see that initial velocity of the object is u (at point A) and then it increases to v (at point B) in time t. The velocity changes at a uniform rate a.
           • Again from figure it is clear that time t is represented by OC , initial velocity u by OA and final velocity of object after time t by BC.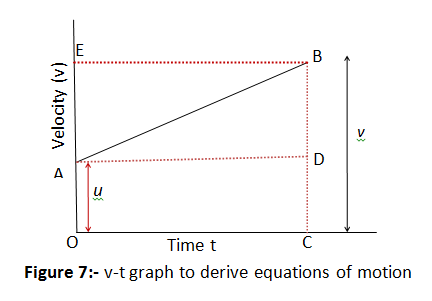
             
           • From graph as given in figure 7 it is clear that BC=BD+DC=BD+OA.
             So we have 
             v=BD+u               (1)
           • We should now find out the value of BD. From the velocity-time graph (Fig. 7), the acceleration of the object is given by
             
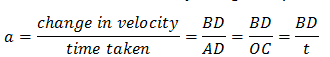
             which gives, BD=at
             putting this value of BD in equation 1 we get
             v=u+at
             which is the equation for velocity time relation.

             b. Equation for position time relation

             • Let us consider that the object has travelled a distance s in time t under uniform acceleration a. In Fig. 7, the distance travelled by the object is obtained by the area enclosed within OABC under the velocity-time graph AB.
             • Thus, the distance s travelled by the object is given by
               s = area OABC (which is a trapezium) 
               s= area of the rectangle OADC + area of the triangle ABD
               So, 
               s=OA×OC+12)AD×BD)
               Substituting OA=u, OC=AD=t and BD=at, we get
               s=(u×t)+12×(t×at)
               or,
               s=ut+12at2
               which is the equation of position time relation

             c. Equation for position velocity relation

             • Again consider graph in figure 7. We know that distance travelled s by a body in time t is given by the area under line AB which is area of trapezium OABC. So we have
               
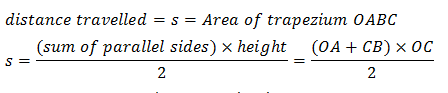
               Since OA+CB=u+v and OC=t, we thus have
               s=(u+v)t2
               From velocity time relation
               t=v−ua
               putting this t in equation for s we get
               s=(u+v)2(v−ua)
               or we have
               v2=u2+2as
               which is equation for position velocity relation.

               9. Uniform circular motion

               • When an object moves in a circular path at a constant speed then motion of the object is called uniform circular motion.
               • In our everyday life ,we came across many examples of circular motion for example cars going round the circular track and many more .Also earth and other planets revolve around the sun in a roughly circular orbits
               • If the speed of motion is constant for a particle moving in a circular motion still the particles accelerates because of constantly changing direction of the velocity.
               • If an object moves in a circular path with uniform speed, its motion is called uniform circular motion
               • Here in circular motion, we use angular velocity in place of velocity we used while studying linear motion.
               • Force which is needed to make body travel in a circular path is called centripetal force.
               • We know that the circumference of a circle of radius r is given by 2πr. If the body takes t seconds to go once around the circular path of radius r, the velocity v is given by
                 v=2πrt
               • One thing we must keep in mind is that uniform linear motion is not accelerated but uniform circular motion is accelerated motion.
               • Examples of uniform circular motion are 
                 (a) Motion of artificial satellites around the earth
                 (b) Moon, the natural satellite of earth, moves in uniform circular motion round the earth.
                 (c) Cyclist moving on a circular track with a constant speed exhibits uniform circular motion.